No Function Continuous on Rationals and Discontinuous on Reals
In This Article
-
What is a Continuous Function?
-
What is a Discontinuous Function?
-
Properties of Continuous Functions
-
Theorems for Continuous Functions
What is a Continuous Function?
A function is continuous everywhere if you can trace its curve on a graph without lifting your pencil. A function is discontinuous at a point if you cannot trace its curve without lifting your pencil at that point; meaning it has a hole, break, jump, or vertical asymptote at that point.
For example, the function is continuous everywhere. We can draw its curve without ever lifting our hand. By contrast, the function has a discontinuity at . We can't draw its curve without lifting our pencil at .
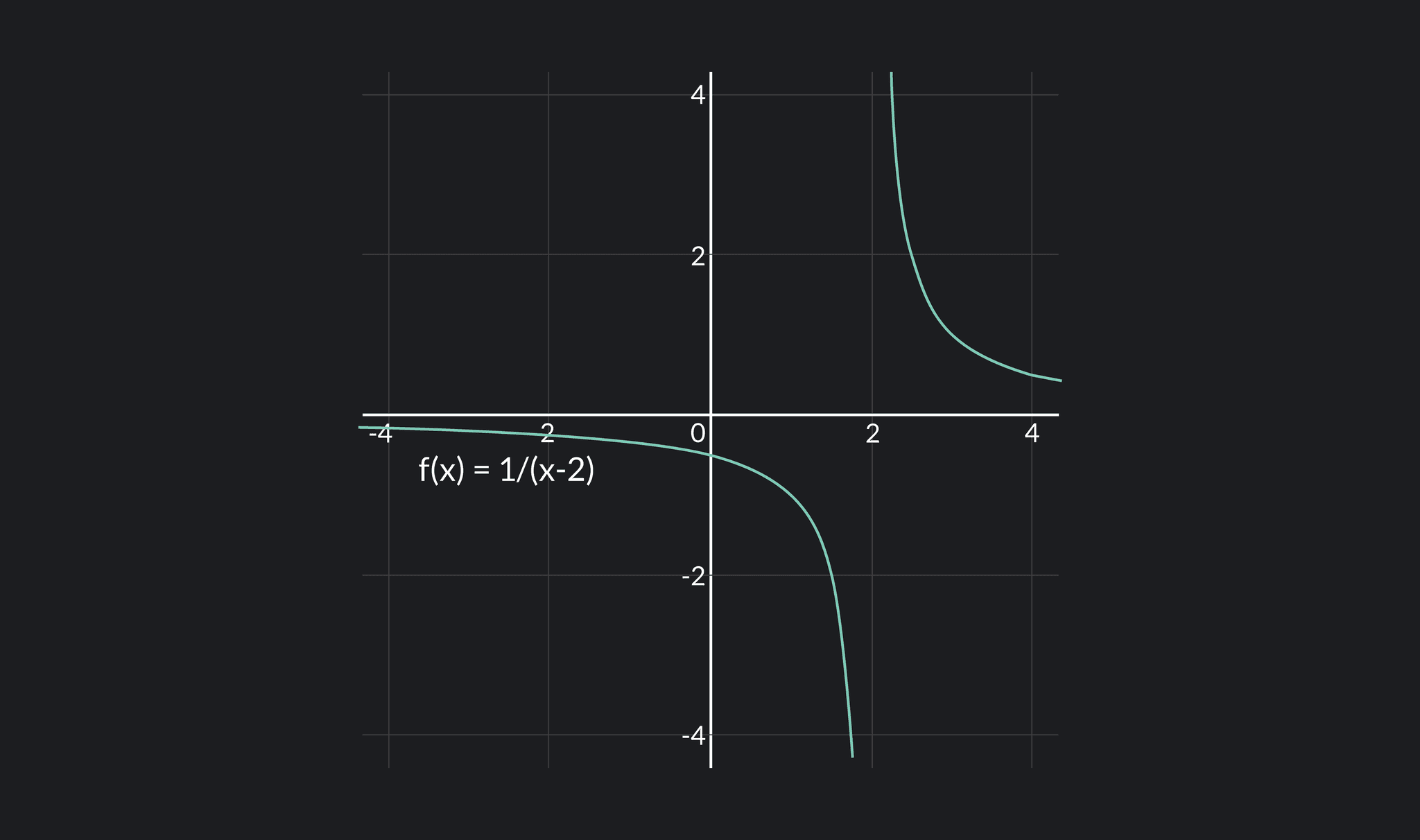
In differential calculus, it's important to understand the concept of continuity because functions that are not continuous are not differentiable.
Let's learn how to prove a function is continuous at a point. Here's the formal definition of continuity at a point.
A function is continuous at the point if:
-
exists
-
exists
-
In order to show that a function is continuous at a point , you must show that all three of the above conditions are true. To refresh your knowledge of evaluating limits, you can review How to Find Limits in Calculus and What Are Limits in Calculus.
For example, let's show that is continuous at .
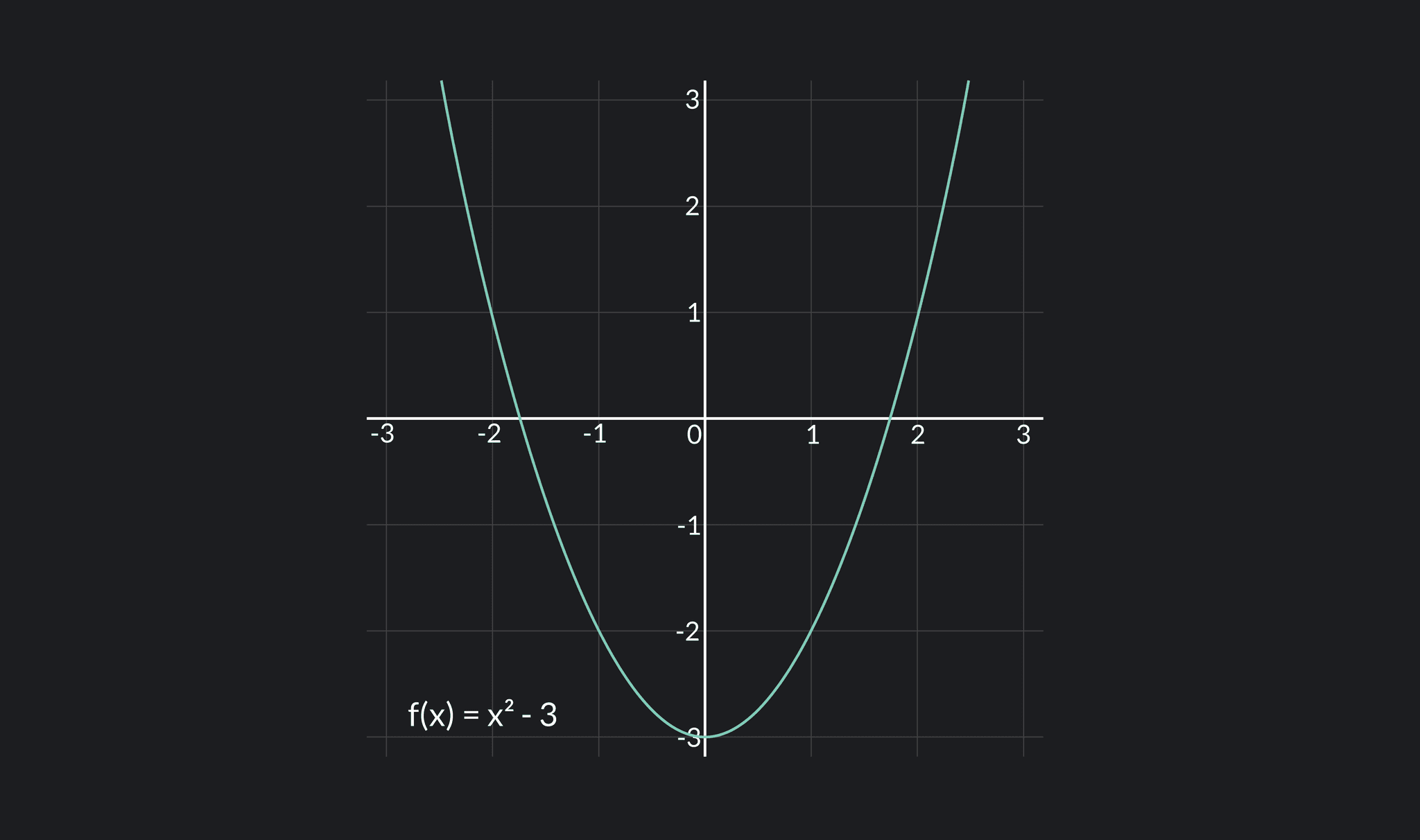
Thus, is continuous at . In fact, we could show that is continuous everywhere.
Other functions might be continuous only over a specific interval of the real numbers. If a function is continuous on an open interval, that means that the function is continuous at every point inside the interval.
For example, has a discontinuity over the real numbers at , since we must lift our pencil in order to trace its curve. However, we can say that is continuous on the open interval since it is continuous at every point inside that specific interval.
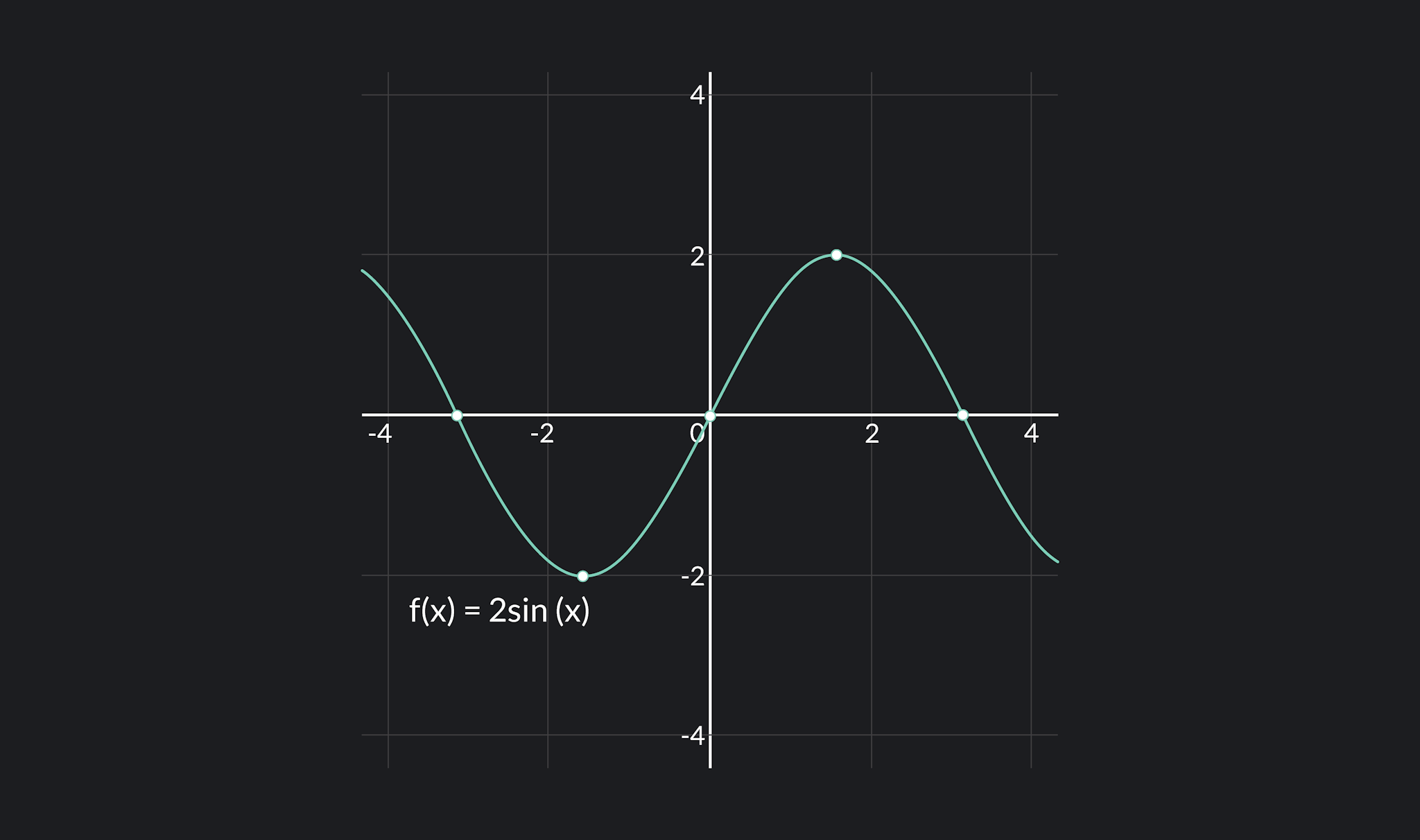
We can also say that is continuous over its own domain, which is any real number excluding odd multiples of .
What is a Discontinuous Function?
A discontinuity is a hole, jump, break, or vertical asymptote on a function's curve.
There are 3 types of discontinuities.
Jump Discontinuities
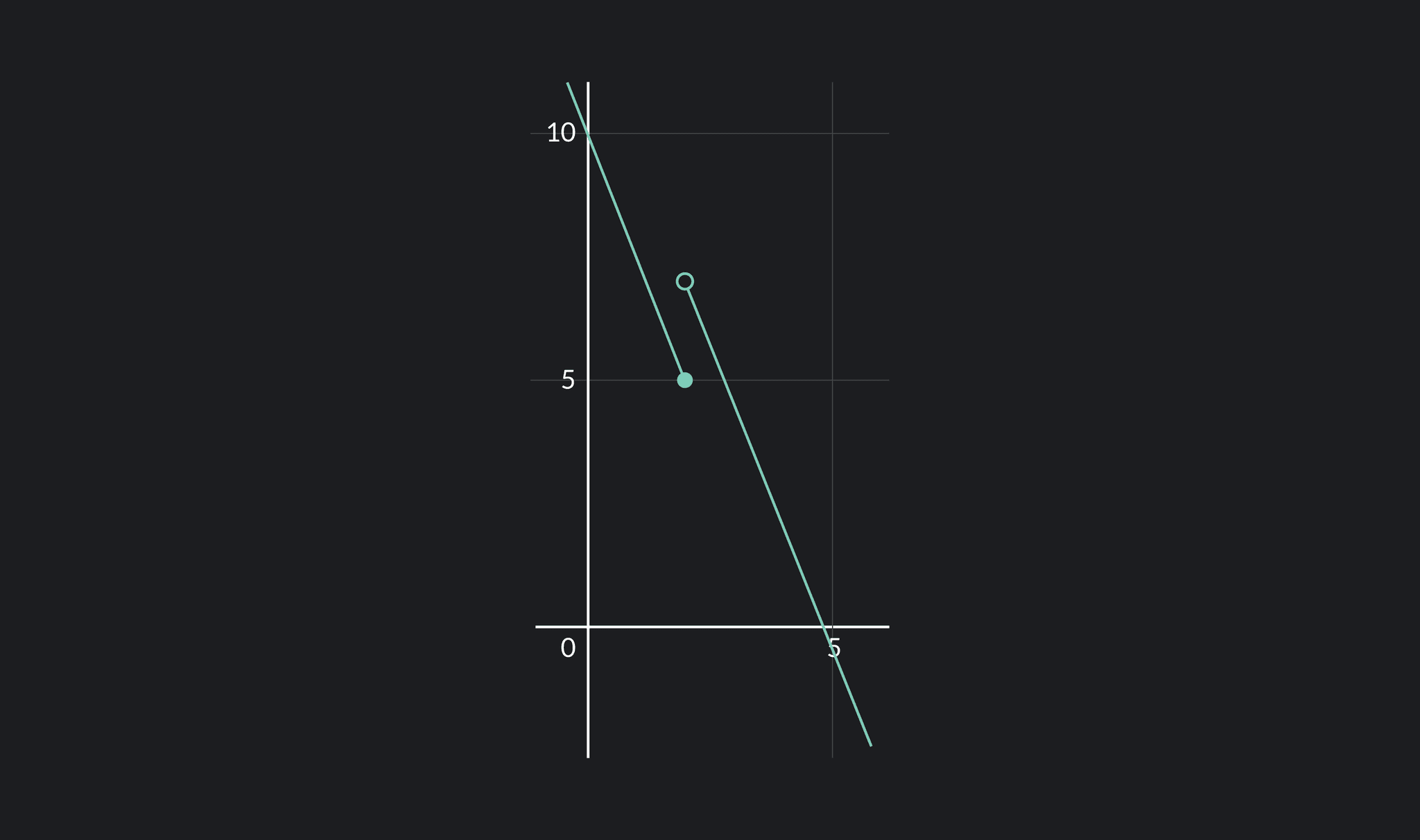
If a function has a jump discontinuity at some point , then does not exist. Remember that in order for a limit to exist, its one-sided limits must exist and they must equal the same value. In other words, the limit as approaches from the left must equal the limit as approaches from the right.
In functions with jump discontinuities, . For example, in the function above, and . Thus, does not exist, and so there is a discontinuity at .
Removable Discontinuities
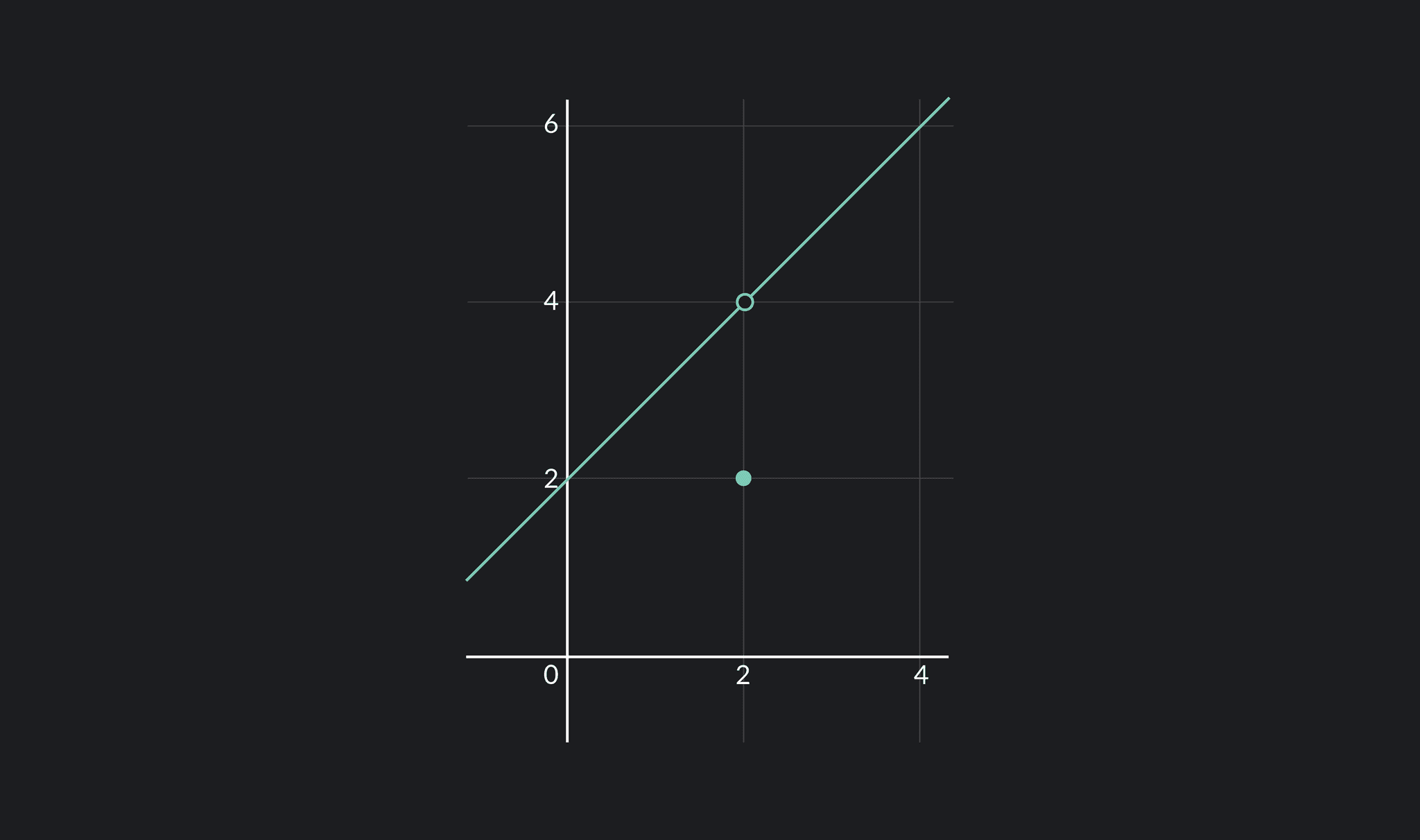
If a function has a removable discontinuity at some point , then . On a graph, this looks like a hole. In these discontinuities, the one-sided limits as approaches always equal each other. However, the function's value at equals something different or might not exist at all.
For example, in the function above, . However, . Since , the function has a discontinuity at .
Infinite Discontinuities
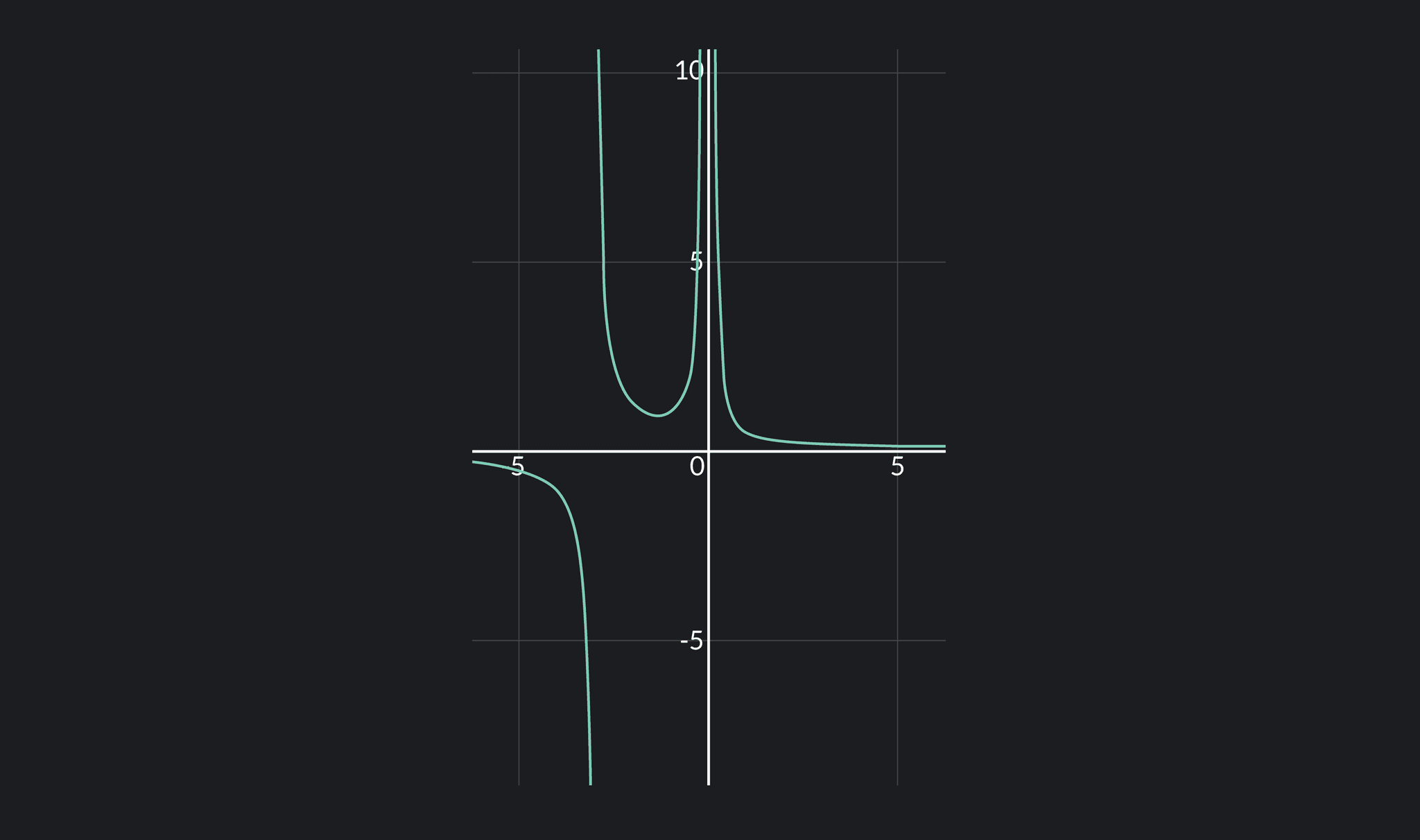
If a function has an infinite discontinuity at some point , then the function has a vertical asymptote at . If any one of the following statements are true, then has a vertical asymptote at .
For example, in the function above, there is a vertical asymptote at and . Thus, there is an infinite discontinuity at and .
Properties of Continuous Functions
If and are both continuous at , then the following properties are true:
-
The sum is continuous at .
-
The difference is continuous at .
-
The product is continuous at .
-
The quotient is continuous, provided .
-
The constant multiple is continuous at for any number .
-
The composition is continuous at if is continuous at .
Theorems for Continuous Functions
Extreme Value Theorem
The Extreme Value Theorem states that if a function is continuous on the closed interval , then the function must have both a maximum and a minimum on .
Intermediate Value Theorem
The Intermediate Value Theorem is an extremely useful theorem in math. It's often used to prove that different equations are solvable. It's especially useful for proving that a function has a root on a particular interval. The root of a function is the point at which a function equals zero and crosses the x-axis. The Intermediate Value Theorem states:
Suppose
More simply, the Intermediate Value Theorem says that a continuous function must take on every value between and at least once on the interval .
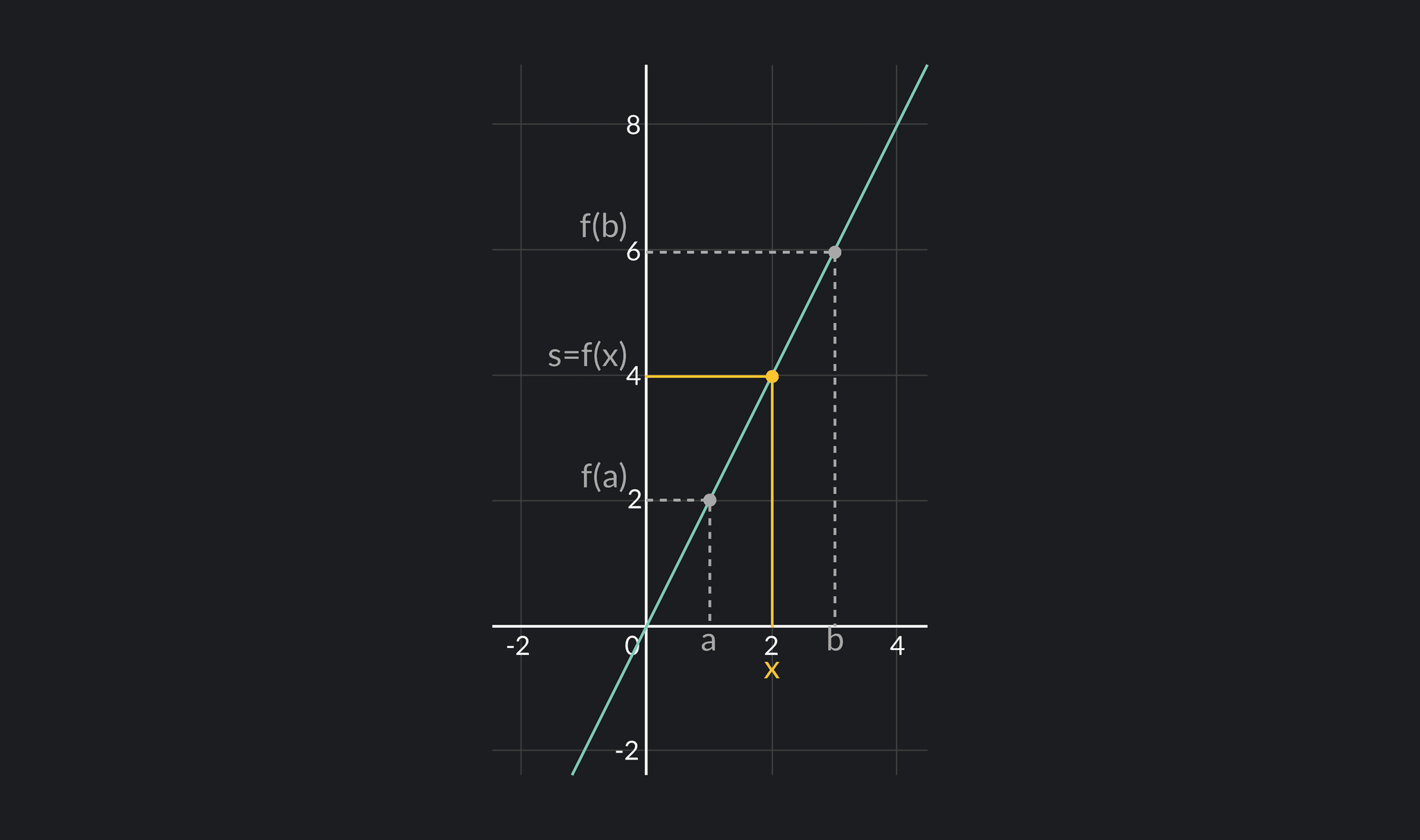
For example, consider the graph of in the above graph. Let's examine the interval where and . Since and , we'll choose an in-between value for our s-value.
Then, since is continuous on , the Intermediate Value Theorem guarantees that there must exist some on such that . By looking at the graph, we can see that is the value for which .
Polynomial Function
Any polynomial function is continuous for all real numbers. A polynomial function is a function consisting of variables and coefficients that involves only non-negative exponents of the variable. Polynomials use only addition, subtraction, and multiplication operations. For example, is a polynomial function.
Differentiable Function
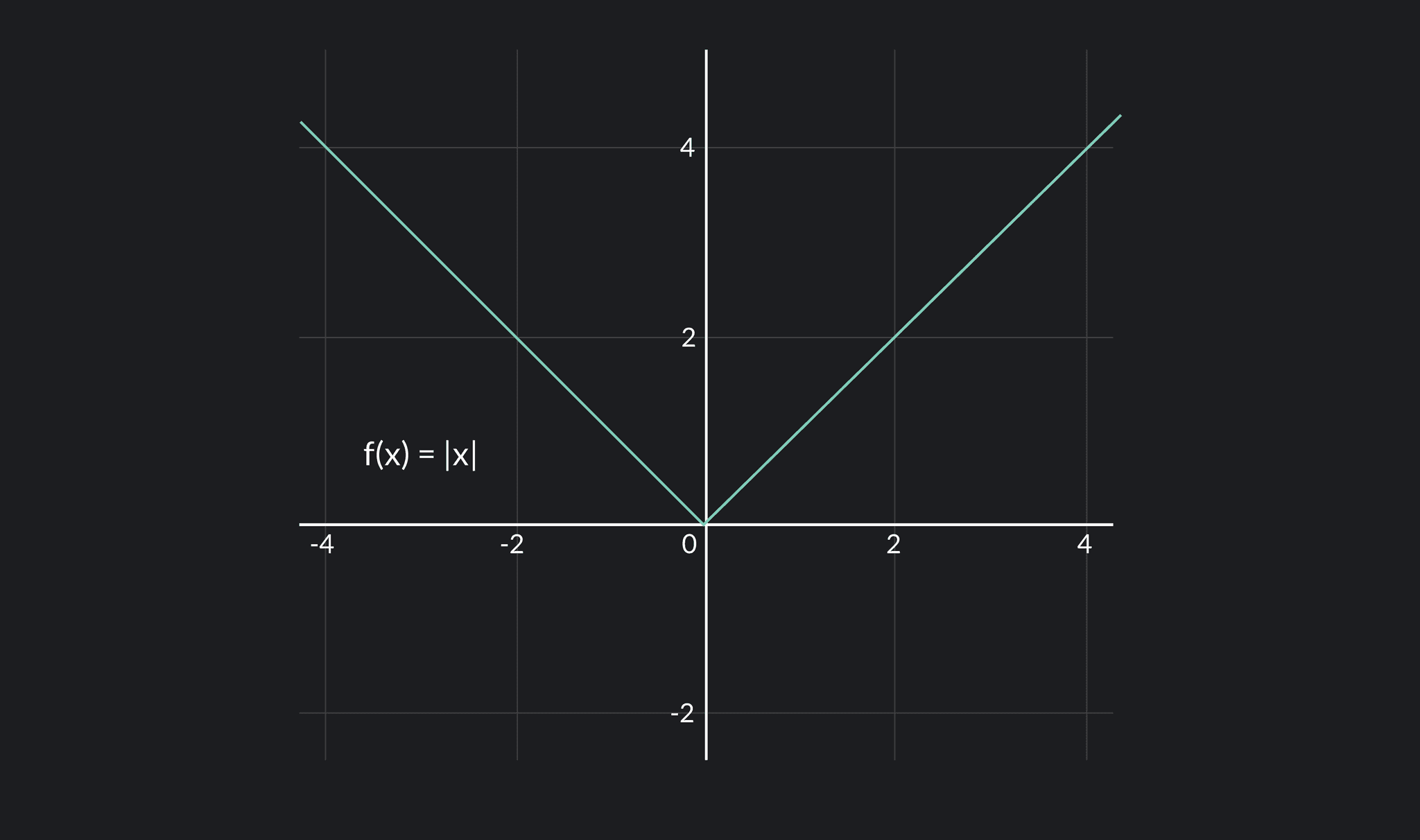
Every differentiable function is continuous. However, be careful to remember that the converse is not necessarily true. A function could be continuous, but not differentiable. For example, the absolute value function below is continuous at but not differentiable at .
Other Functions
Rational, root, trigonometric, exponential, and logarithmic functions are all continuous in their domains. The domain of a function is the set of values that a function can accept as inputs. Many real life examples of continuous functions can be modeled using these function types.
Rational Functions
A rational function is a function that is written as the ratio of two polynomial functions. The domain of rational functions is all numbers except those that make the denominator zero. So, the values where rational functions have vertical asymptotes or removable discontinuities are outside of their domain.
Trigonometric Functions
The trigonometric functions and have domains that include all real numbers. So, they are continuous for all real numbers.
Other trigonometric functions such as $\tan(x)$ have more selective domains — for example, the domain of is equal to all real numbers except for where . This occurs at every odd multiple of , and so these x-values are outside the domain of .
Exponential Functions
Exponential functions have the form , where and is a real number greater than 1. The domain of exponential functions is all real numbers.
Logarithmic Functions
Logarithmic functions are only defined for positive inputs. So, the domain of logarithmic functions can be determined by solving the inequality that sets the inside terms to be greater than 0.
Explore Outlier's Award-Winning For-Credit Courses
Outlier (from the co-founder of MasterClass) has brought together some of the world's best instructors, game designers, and filmmakers to create the future of online college.
Check out these related courses:
Source: https://articles.outlier.org/continuous-function-definition
0 Response to "No Function Continuous on Rationals and Discontinuous on Reals"
Post a Comment